Answer:
The margin of error is of 0.17.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.

In which
z is the zscore that has a pvalue of
 .
.
The margin of error is:

He surveyed 35 mortgage holders and found that the proportion of these that did expect to own their house within 10 years is 0.51.
This means that
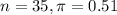
95% confidence level
So
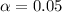 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
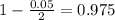 , so
, so
 .
.
a)Calculate the margin of error that the high school student will have.

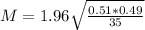
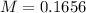
Rounding to 2 decimal places, the margin of error is of 0.17.