Answer:
1. 3413
2. 4886
3. 114
Explanation:
Normal Probability Distribution:
Problems of normal distributions can be solved using the z-score formula.
In a set with mean
 and standard deviation
and standard deviation
 , the z-score of a measure X is given by:
, the z-score of a measure X is given by:

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.
Mean is 400 hours and the standard deviation is 50 hours
This means that
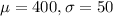
1. Between 350 and 450 hours:
The proportion is the pvlaue of Z when X = 450 subtracted by the pvalue of Z when X = 350.
X = 450:

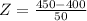
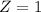
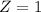 has a pvalue of 0.8413.
has a pvalue of 0.8413.
X = 350:

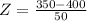
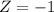
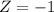 has a pvalue of 0.1587.
has a pvalue of 0.1587.
0.8413 - 0.1587 = 0.6826
Out of 5000:
0.6826 out of 5000. So
0.6826*5000 = 3413
3413 are expected to last between 350 hours and 450 hours.
2.more than 300 hours
The proportion is one subtracted by the pvalue of Z when X = 300.

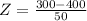
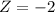
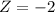 has a pvalue of 0.0228
has a pvalue of 0.0228
1 - 0.0228 = 0.9772
Out of 5000:
0.9772of 5000 is
0.9772*5000 = 4886
4886 are expected to last more than 300 hours.
3.less than 300 hours
4886 are expected to last more than 300 hours, so 5000 - 4886 = 114 are expected to last less than 300 hours.