Question

The formula for throwing a baseball in the air is represented by h=-16t^2 + 12t + 40 where h is the height of the ball. What is the maximum height of the ball?

Answer 1

the maximum height of the ball is 42.25 m

Explanation:

Given the height function as;

h(t) = -16t² + 12t + 40

At maximum height, the final velocity of the ball will be zero.

The final velocity is calculated as follows;

`v = (dh(t))/(dt) = -32 t+ 12\\\\at \ maximum \ height\ v = 0\\\\Thus, -32 t+ 12 = 0\\\\32t = 12\\\\t = (12)/(32) \\\\t = 0.375 \ s`

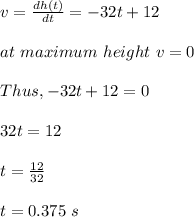
At maximum height, the time of motion of the ball is 0.375 s.

The maximum height is calculated as follows;

h(t) = -16t² + 12t + 40

h(0.375) = -16(0.375)² + 12(0.375) + 40

h(0.375) = -2.25 + 4.5 + 40

h(0.375) = 42.25 m

Therefore, the maximum height of the ball is 42.25 m