Question

Helppppp

The sides of a triangle measure 7, 4, and 9. If the longest side of a similar triangle measures 36, determine and state the length of the shortest side of this triangle.


The aspect ratio (the ratio of screen width to height) of a rectangular flat-screen television is 16 : 9. The length of the diagonal of the screen is the television's screen size. Determine and state, the width of the flat-screen television if the height is 20.6 inches. Round your answer to the nearest hundredth.

Answer 1

1. 16

2. 36.62 in

Explanation:

For the first one:

If you don't want to read the explanation go to the next section in bold

We are told that the triangles are similar.

We are told that the side lengths of the smaller triangle measure 7,4 and 9

And we are told that the longest side of the similar triangle measures 36

Given this information we need to find the other side lengths.

Because the triangles are similar each side length will share similar ratios. Note that the ratios must be between the two longest side, the two medium length sides and the two shorter sides

Because we are given the longest side length of the similar triangle and the longest side length of the smaller triangle we can set up a proportion to find the other side lengths.

The proportion can be found by dividing the longest side length of the larger triangle (36) by the longest side length of the smaller triangle (9)

proportion =
`(36)/(9) or4`

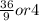
So the ratio to the side lengths of the triangle is 4 to 1

Now that we have created a proportion we can find the other side lengths.

Once again this information is not needed. If you don't want to read it go to the next section in bold.

For the medium length side:
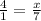`(4)/(1) =(x)/(7)` ( remember x = missing side length)

multiply each side by 7

4 * 7 = 28

x/7 * 7 = x

x = 28

For the shorter side:
`(4)/(1) =(x)/(4)` ( once again x = missing side length )

multiply each side by 4

4 * 4 = 16

x/4 * 4 = x

x = 16

So we can conclude that the side length of the shortest side is 16

For the second one

This problem is like the other one.

The only difference is we are already given the ratio

( note that the ratio is width to height )

16 : 9

Given the proportion and the height (20.6) of the other flat screen tv we need to find the width

To do so we set up an equation using the ratio:

let x = width

`(16)/(9) =(x)/(20.6)`

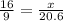
using cross multiplication

16 * 20.6 = 329.6

9 * x = 9x now we have 9x = 329.6

step 2 divide each side by 9

9x / 9 = x

329.6/9 = 36.62

we're left with x = 36.62

Meaning that the width of the other flat screen tv is 36.62 in