Answer:
The possible values of
 are -2.944 and -9.055, respectively.
are -2.944 and -9.055, respectively.
Explanation:
From statement we know that
 . By Analytical Geometry, we use the equation of a line segment, which is an application of the Pythagorean Theorem:
. By Analytical Geometry, we use the equation of a line segment, which is an application of the Pythagorean Theorem:

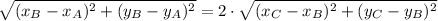 (1)
(1)
Where:
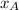 ,
,
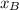 ,
,
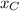 - x-Coordinates of points A, B and C.
- x-Coordinates of points A, B and C.
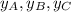 - y-Coordinates of points A, B and C.
- y-Coordinates of points A, B and C.
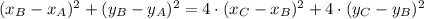
Then, we expand and simplify the expression above:
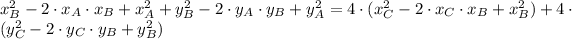
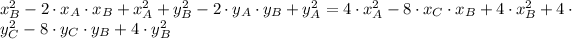
If we know that
 ,
,
 ,
,
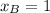 ,
,
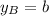 ,
,
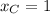 and
and
 , then we have the following expression:
, then we have the following expression:
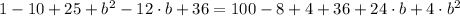
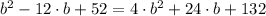
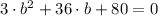
This is a second order polynomial, which means the existence of two possible real solutions. By Quadratic Formula, we have the following y-coordinates for point B:
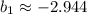 ,
,
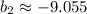
In consequence, the possible values of
 are -2.944 and -9.055, respectively.
are -2.944 and -9.055, respectively.