Answer:
Explanation:
Quadratic equation has been given as,
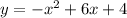
Converting this equation into vertex form,
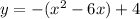
![y=-[x^(2)-2(3x)+9-9]+4](https://img.qammunity.org/2022/formulas/mathematics/college/kuwxo5y7935g7zk2qufsfjltgj72rb0tgc.png)
![y=-[x^(2)-2(3x)+3^2]+4+3^2](https://img.qammunity.org/2022/formulas/mathematics/college/mli5ldhmn30200tur86lolhpxm2eso4ky6.png)


By comparing this equation with the vertex form of a quadratic equation,
y = (x - h)² + k
Here, (h, k) is the vertex of the parabola.
Vertex of the parabola → x = 3, y = 13
Axis of symmetry → x = 3