Answer:
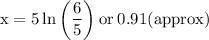
Explanation:
we are given a logarithmic equation
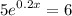
we want to figure out x
remember that,

therefore to use the above formula with our given equation
divide both sides by 5:
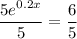
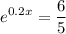
take In both sides:
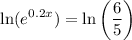
by using the formula we acquire
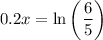
we can rewrite left hand side as fraction
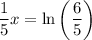
multiply both sides by 5
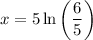
and we are done!