Question

The height in feet of a baseball can be modeled by the function y=-16t^2+64t where t is the time in

seconds after the ball is hit. Find the baseball's maximum height and the time it takes to reach this
height. Then find how long the baseball is in the air.

Answer 1

the ball reaches a height of 64 feet after 2 sec.

The ball is in the air 4 sec.

Explanation:

The ball is following the path of a parabola. The maximum height is at the vertex of the parabola.

Let (h, k) be the vertex. h will be the time it takes to reach the maximum height, and k will be that height.

y =
`-16t^(2) + 64t`

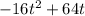
h = -b/2a = 64/(-2)(-16) = 64/32 = 2

k = -16(2)^2 + 64(2)

-16(4) + 128

- 64 + 128 = 64

V: (2, 64)

So the ball reaches a height of 64 feet after 2 sec.

Let
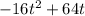`-16t^(2) + 64t` = 0 (0 is the height when the balls hits the ground)

-16t(t - 4) = 0

t = 0 or t = 4

The ball is in the air 4 sec.