Answer:
I) ∠CAB measures 60°.
II) BC measures approximately 15.23 cm.
*Please read notes.
Explanation:
We are given ΔABC, where AB measures 10√3 cm, AC measures 6 cm, and the triangle has an area of 45 square cm.
And we want to find I) the measure of ∠CAB and II) the length of BC.
I) First, we should always draw a representative triangle so we can determine the sides and angles. This is shown below.
Note that ∠CAB (or simply ∠A) is the angle between the two sides. Thus, we can find the angle by using the alternative formula for the area of a triangle:
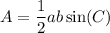
Where a and b are two side lengths, and C is the angle between the two sides.
Substitute:

Simplify:
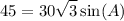
So:
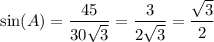
Take the inverse sine of both sides. Use a calculator. Thus:
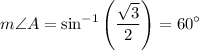
II) To find BC, we will use the Law of Cosines. We do not know whether or not ΔABC is a right triangle, so we cannot use right triangle trigonometry or special right triangles.
The law of cosines is:
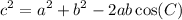
Where a and b are side lengths and C is the angle between the side lengths. c is the side length opposite to the angle.
BC is opposite to A. Substitute:
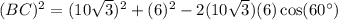
Simplify:
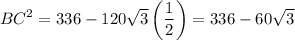
Take the square root of both sides:
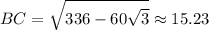
BC measures about 15.23 cm.
Notes:
In this case, the inverse sine of √3/2 will yield two answers: 60° and 120°. This is an example of an ambiguous case. Both of these angles will work. However, BC will be different. If ∠A is 60°, then BC is about 15.23. However, if ∠A is 120°, then BC is about 20.97. Both will result in the triangle having an area of 45 square cm, as well as AB measuring 10√3 and AC measuring 6.