Question

Write the standard form of the equation of the circle with center (4,7) that passes through the point (−7,9).

Answer 1

`(x-4)^2+(y-7)^2=125`

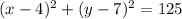
Explanation:

1) Find the radius

To find the radius of the circle, use the distance formula for the points (4,7) and (-7,9)

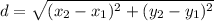`d=√((x_2-x_1)^2+(y_2-y_1)^2)` where the two points are
`(x_1,y_1)` and
`(x_2,y_2)`

Plug in the points (4,7) and (-7,9)

`d=√((-7-4)^2+(9-7)^2)\\d=√((-11)^2+(2)^2)\\d=√(121+4)\\d=√(125)`

Therefore, the radius of the circle is
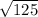`√(125)` units.

2) Plug all data into the circle equation

Equation of a circle (not centred at the origin):

`(x-h)^2+(y-k)^2=r^2` where the centre is
`(h,k)`

Plug in the point (4,7) as (h,k)

`(x-4)^2+(y-7)^2=r^2`

Plug in the radius
`√(125)`

`(x-4)^2+(y-7)^2=(√(125)) ^2\\(x-4)^2+(y-7)^2=125`

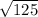
I hope this helps!