Can someone help with this please????

Question

asked Jun 21, 2022 592 views

1 Answer

RobertF · Answer 1 · 2022-06-28T01:26:10+0000

Given:

The given function is:

f(x)=11x-11

To find:

The value of
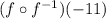$(f\circ f^(-1))(-11)$ .

Solution:

We have,

f(x)=11x-11

First we have to find the inverse of this function.

Putting f(x)=y, we get

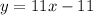
y=11x-11

Interchange x and y.

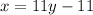
x=11y-11

Isolate the variable y.

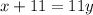
x+11=11y

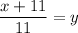
(x+11)/(11)=y

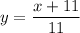
y=(x+11)/(11)

Putting
y=f^(-1)(x) , we get

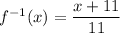
f^(-1)(x)=(x+11)/(11)

Now,

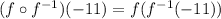
$(f\circ f^(-1))(-11)=f(f^(-1)(-11))$

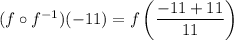
$(f\circ f^(-1))(-11)=f\left((-11+11)/(11)\right)$
$\left[\because f^(-1)(x)=(x+11)/(11)\right]$

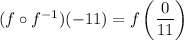
$(f\circ f^(-1))(-11)=f\left((0)/(11)\right)$

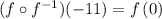
$(f\circ f^(-1))(-11)=f\left(0\right)$

The given function is
f(x)=11x-11 .

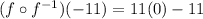
$(f\circ f^(-1))(-11)=11(0)-11$

$(f\circ f^(-1))(-11)=-11$

Therefore, the value of
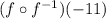$(f\circ f^(-1))(-11)$ is
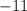. Hence the correct option is A.