Question

A rocket is launched from a tower. The height of the rocket, y in feet, is related to the

time after launch, x in seconds, by the given equation. Using this equation, find the
maximum height reached by the rocket, to the nearest tenth of a foot.
y = -16x2 + 125x + 147

Answer 1

The maximum height of the rocket is about 391.1 feet.

Explanation:

The height of the rocket y in feet x seconds after launch is modeled by the equation:

`y=-16x^2+125x+147`

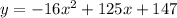
We want to find the maximum height reached by the rocket.

Since this is a quadratic equation, the maximum height occurs at the vertex. The vertex of a quadratic is given by:

`\displaystyle \left(-(b)/(2a), f\left(-(b)/(2a)\right)\right)`

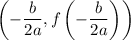
In this case, a = -16, b = 125, and c = 147.

Thus, the x-coordinate of our vertex is:

`\displaystyle x=-(125)/(2(-16))=(125)/(32)`

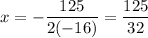
To find the maximum height, we will substitute this value back in. So:

`\displaystyle y_{\text{max}}=-16\left((125)/(35)\right)^2+125\left((125)/(32)\right)+147\approx391.1\text{ feet}`

The maximum height of the rocket is about 391.1 feet.