Question

A sample of size 168, taken from a normally distributed population whose standard deviation is known to be 8.60, has a sample mean of 80.60. Suppose that we have adopted the null hypothesis that the actual population mean is equal to 80, that is, H0 is that μ = 80 and we want to test the alternative hypothesis, H1, that μ ≠ 80, with level of significance α = 0.1. The upper limit of a 95% confidence interval for the population mean would equal: _______

Answer 1

The 95% confidence interval for the population mean is

(79.2996, 81.9004)

Explanation:

Step(i):-

Given that the sample size 'n' = 168

Let 'X' be a Random variable in a normal distribution

Given that mean of the sample x⁻ = 80.60

Given that the standard deviation of the Population = 8.60

Step(ii):-

The 95% confidence interval for the population mean is determined by

`(x^(-) - Z_(0.05) (S.D)/(√(n) ) ,x^(-) + Z_(0.05) (S.D)/(√(n) ) )`

`(80.60 -1.96 (8.60)/(√(168) ) ,80.60 + 1.96 (8.60)/(√(168) ) )`

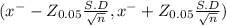
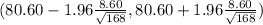
(80.60 -1.3004 , 80.60+1.3004)

(79.2996 , 81.9004)

Final answer:-

The 95% confidence interval for the population mean is

(79.2996, 81.9004)