Question

On a coordinate grid, a trapezoid has vertices at (−6, 9), (−6, 6), (3, −9), and (3, 12). Each square on the grid represents 1 square foot. Explain how to find the area of the trapezoid.

Answer 1

The area of the trapezoid is 108 square feet

Explanation:

The given vertices of the trapezoid are;

(-6, 9), (-6, 6), (3, -9, and (3, 12)

Let the letters A, B, C, and D represent the vertices of the trapezoid, we have;

A(-6, 9), B(-6, 6), C(3, -9) and D(3, 12)

The drawing of the trapezoid on the coordinate plane created with MS Excel is attached

The area of a trapezoid,
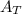`A_T` = (a + b)×h/2

Where;

'a', and 'b' = The length of the bases (parallel sides)

h = The height of the trapezoid (The distance between the bases)

Let 'a' represent the length of the long base CD and let 'b' represent the length of the short base AB

a = √((-9 - 12)² + (3 - 3)²) = 21

∴ a = 21 feet

Similarly, we have;

b = √((9 - 6)² + (-6 - (-6))² = 3

The height, h = The distance between AB and CD = AE

We note that sides AB and CD are parallel to the y-axis, therefore, the height which is the perpendicular distance to the between AB and CD will be parallel to the x-axis

Therefore, the t-coordinates of the points A and E are both equal to 9 and the length of AE = √((3 - (-6))² + (9 - 9)²) = 9

The height of the trapezoid, AE = 9 feet

∴ The area of the trapezoid, A = (21 + 3) × 9/2 = 108

The area of the trapezoid,
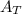`A_T` = 108 ft.²