Question

Dorothy Little purchased a mailing list of 2,000 names and addresses for her mail order business, but after scanning the list she doubts the authenticity of the list. She randomly selects five names from the list for validation. If 40% of the names on the list are non-authentic, and x is the number on non-authentic names in her sample, the expected (average) value of x is ______________.

a) 2.50
b) 2.00
c) 1.50
d) 1.25
e) 1.35

Answer 1

b) 2.00

Explanation:

For each name, there are only two possible outcomes. Either it is authentic, or it is not. The probability of a name being authentic is independent of any other name. This means that we use the binomial probability distribution to solve this question.

Binomial probability distribution

Probability of exactly x sucesses on n repeated trials, with p probability.

The expected value of the binomial distribution is:

`E(X) = np`

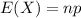
She randomly selects five names from the list for validation.

This means that
`n = 5`

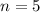
40% of the names on the list are non-authentic

This means that
`p = 0.4`

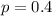
The expected (average) value of x is

`E(X) = np = 5*0.4 = 2`

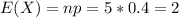
The correct answer is given by option B.