Given:
The graph of the system of inequalities.
To find:
The system of inequalities.
Solution:
From the given graph it is clear that the first line passes through the points (0,2) and (1,-1). So, the equation of the boundary line is:
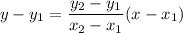
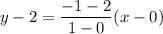
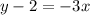
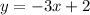
The shaded region lies above the line and the boundary line is a solid line. So, the sign of inequality must by ≥.
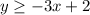
The second line passes through the points (0,-2) and (3,0). So, the equation of the boundary line is:
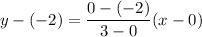


The shaded region lies above the line and the boundary line is a solid line. So, the sign of inequality must by ≥.
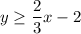
Therefore, the system of inequalities contains two linear inequalities
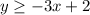 and
and
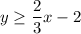 .
.