Answer:
a) The golf ball reaches a height of 64 feet.
b) The golf ball will take 2 seconds to reach maximum height.
c) The golf ball will take 4 seconds to land after being hit.
d) The golf ball will be 48 feet above ground 1 and 3 seconds after being hit.
Explanation:
a) The parabolic motion of the golf ball is described by a second order polynomial, that is, the equation of the parabola. To determine the maximum height of the golf ball, we need to transform the equation of the parabola given into vertex form:

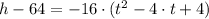
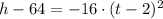
From this form we can obtain relevant information of the maximum height of the golf ball, contained in the left side of the equation. On this approach, we conclude that the golf ball reaches a height of 64 feet.
b) The time taken by the golf ball is contained in the right side of the formula. That is, the golf ball will take 2 seconds to reach maximum height.
c) In this case, we need to factor the polynomial to find right times, that is:
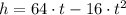
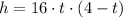
The time taken by the golf ball to land is contained in the second binomial. In a nutshell, the golf ball will take 4 seconds to land after being hit.
d) If we know that
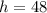 , the time taken by the golf ball to be 48 feet above the ground is:
, the time taken by the golf ball to be 48 feet above the ground is:
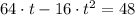
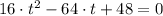
By Quadratic Formula, we have the following roots:
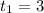 ,
,
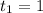
The golf ball will be 48 feet above ground 1 and 3 seconds after being hit.