Answer:
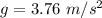
Step-by-step explanation:
Given that,
The length of a simple pendulum, l = 2.2 m
The time period of oscillations, T = 4.8 s
We need to find the surface gravity of the planet. The time period of the planet is given by the relation as follows :
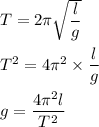
Put all the values,
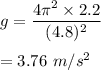
So, the value of the surface gravity of the planet is equal to
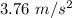 .
.