Question

) Cars are parked in line as they come off the assembly lines. There are three models: red cars which take up 2 spaces, blue cars which also take up 2 spaces, and green cars which take up only 1 space. Let an be the number of ways of filling the first n parking spaces with red, blue and green cars.

Answer 1

Hello your question has some missing information below is the complete question

answer :

`a_(n) = a_(n-2) + a_(n-2) + a_(n-1)`

Explanation:

Red car and Blue car take up 2 spaces each

Green cars take up 1 space

lets determine the number of ways of filling up the parking spaces

i) First lets assume the last space is filled by Green car then there will be
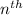`n^(th)` space occupied hence there will be ( n - 1 ) spaces left to be filled in
`a_(n-1)` ways

ii) lets assume the last space is filled by either a Red car or a Blue car then there will be
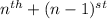`n^(th) + ( n -1 )^(st)` parking space occupied hence there will be

( n - 2 ) spaces left to be filled in
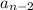`a_(n-2)` ways

Hence A closed formula for
`a_(n)`

`a_(n) = a_(n-2) + a_(n-2) + a_(n-1)`

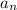
where : a1 = 1 way , a2 = 3 ways