Answer:
The 98% of the confidence interval for the true average salary of Knirhsdaeh employees as a psychology counselor
(75.4206, 86.5794)
Explanation:
Step(i):-
Given that the mean of the sample = $81 k
Given that the size of the sample 'n' = 23
Given that the standard deviation for the salaries is $13 k
Step(ii):-
98% of the confidence interval for the true average salary of Knirhsdaeh employees is determined by

Degrees of freedom = n-1 = 23-1 =22
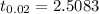
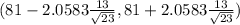
( 81 - 5.57940 , 81 + 5.57940)
(75.4206, 86.5794)
Final answer:-
The 98% of the confidence interval for the true average salary of Knirhsdaeh employees as a psychology counselor
(75.4206, 86.5794)