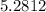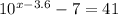
Use the rules of exponents and logarithms to solve the equation.
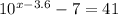
Add 7 to both sides of the equation.

Take the logarithm of both sides of the equation.
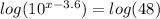
The logarithm of a number raised to a power is the power times the logarithm of the number.
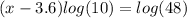
Add 3.6 to both sides of the equation.
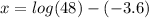
Solve by first finding
 then subtract
then subtract
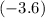 from the answer:
from the answer:
1.
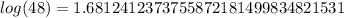
Now just solve the equation:
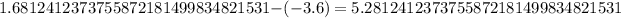
Your answer is C.
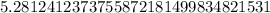 or
or