Question

PLEASE HELP ME SOLVE THIS.

STEP-BY STEP
THANK YOU !!!

I really don't know how to calculate this question.

(3 tan 45°)(4 sin 60°)-(2 cos 30°)(3 sin 30°)
​

Answer 1

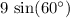`9\,\sin(60^\circ)`, which is equal to
`\displaystyle (9√(3))/(2)`.

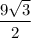Explanation:

An angle of
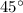`45^\circ` corresponds to an isosceles right triangle: the length of the two legs (adjacent and opposite) would be equal. Accordingly:

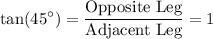`\displaystyle \tan(45^\circ) = \frac{\text{Opposite Leg}}{\text{Adjacent Leg}} = 1`.

Let
`A` denote the measure of an angle. Double-angle identity for sine:

`2\, \sin(A) \cdot \cos(A) = \sin(2\, A)`.

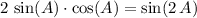By this identity:

`\begin{aligned}& (2\, \cos(30^\circ)) \cdot (3\, \sin(30^\circ)) \\ &= 3\, (2\, \cos(30^\circ) \cdot \sin(30^\circ)) \\ &= 3\, \sin(2 * 30^\circ) \\ &= 3\, \sin(60^\circ)\end{aligned}`.

(
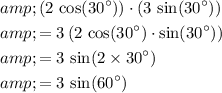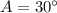`A = 30^\circ` in this instance.)

Hence:

`\begin{aligned}&(3\, \tan(45^\circ)) \cdot (4\, \sin(60^\circ)) - (2\, \cos(30^\circ)) \cdot (3\, \sin(30^\circ)) \\ &= 12\, \sin(60^\circ) - 3\, \sin(60^\circ) \\ &= 9\, \sin(60^\circ)\end{aligned}`.

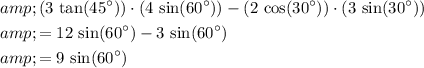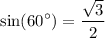`\displaystyle \sin(60^\circ) = (√(3))/(2)`. Therefore,
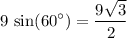`\displaystyle 9\, \sin(60^\circ) = (9 √(3))/(2)`.