Question

A regular reader of BYTE magazine, Fritz, has decided to purchase a computer with these particular specifications. These computers are have an approximate normal distribution with a mean of $850 and a standard deviation of $50. His budget is flexible, and he can afford to spend between $800 and $900. What percentage of these computers are within his budget? (Use the Empirical Rule 68, 95, 99.7)

Answer 1

The probability that can afford to spend between $800 and $900

P(800≤X≤900) = 0.6826

The percentage of that can afford to spend between $800 and $900

P(800≤X≤900) = 68 percentage

Explanation:

Step(i):-

Given that the mean of the Normal distribution = $850

Given that the standard deviation of the Normal distribution = $50

Let 'X' be a random variable in a normal distribution

Let x₁ = 800

`z_(1) = (x_(1)-mean )/(S.D) = (800-850)/(50) = -1`

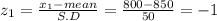
Let x₂ =850

`z_(2) = (x_(2)-mean )/(S.D) = (900-850)/(50) = 1`

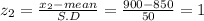
Step(ii):-

The probability that can afford to spend between $800 and $900

P(800≤X≤900) = P(-1≤Z≤1)

= P(Z≤1) - P(Z≤-1)

= 0.5 + A(1) - (0.5 - A(-1))

= A(1) +A(-1)

= 2× A(1) (∵ A(-1) =A(1)

= 2 × 0.3413

= 0.6826

The percentage of that can afford to spend between $800 and $900

P(800≤X≤900) = 68 percentage