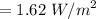Question

A thin beam of light of wavelength 50 μm (in the infrared portion of the em spectrum) goes through

a thin slit and falls on a screen 3.00 m past the slit. You observe that the first completely dark fringes

occur on the screen at distances of ±8.24 mm from the central bright fringe, and that the central bright

fringe has an intensity of I0 at its center. (a) How wide is the slit? (b) What is the highest order fringe

this scenario is capable of producing? (c) What is the intensity of light at a point on the screen that is

one-quarter of the way from the central bright fringe to the first dark fringe?

Answer 1

Given :

Wavelength of the thin beam of light, λ = 50 μm

Distance of the screen from the slit, D = 3.00 m

Width of the fringe, Δy = ±8.24 mm

Therefore, width of the slit is given by :

`$d=(n \lambda D)/(\Delta y)$`

`$=(2 * 50 * 10^(-9) * 3)/(2 * 8.24 * 10^(-3))$`

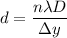
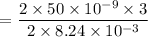
= 0.000018203 m

= 0.0182 mm

= 0.018 mm

The intensity of light is given by :

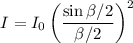`$I=I_0\left((\sin \beta /2)/(\beta/ 2)\right)^2$` , where
`$\beta=(2 \pi D \sin \theta)/(\lambda)$`

`$I=I_0\left((\sin (\pi d \sin \theta)/(\lambda))/((\pi d \sin \theta)/(\lambda))\right)^2$`

`$I=I_0\left((\sin (\pi d y)/(D\lambda))/((\pi d y)/(D\lambda))\right)^2$`

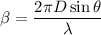
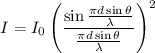
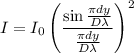
Now,
`$(dy)/(D \lambda) = (8.24 * 10^(-3)* 0.018 * 10^(-3))/(4 * 50* 10^(-9)* 4)$`

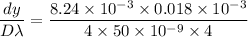
= 0.1854

≈ 0.18

`$I=I_0\left((\sin 0.56)/(0.56)\right)^2$`

`$=I_0 * 0.81$`

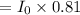
= 2 x0.81

`$= 1.62 \ W/m^2$`