Answer:
Mileage = $71
Loading/Unloading Fee: -$980
Step-by-step explanation:
The question has incomplete details. The complete question can be found online. The given parameters are:
Situation A
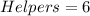
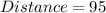
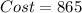
Situation B
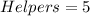
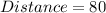

Solving (a): The equation.
Let
x = loading/unloading fee
y = mileage fee
For situation A, the equation will be:
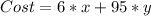
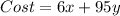

For situation B, the equation will be:

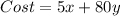
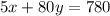
So, the equations are:

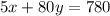
Solving (b): The solution
 --- (1)
--- (1)
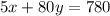 --- (2)
--- (2)
Multiply (1) by 5 and multiply (2) by 6 to eliminate x
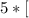

![]](https://img.qammunity.org/2022/formulas/mathematics/middle-school/6k6epemxei0igaktrm2pw36fy23c4xckrh.png)
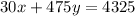
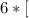
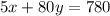
![]](https://img.qammunity.org/2022/formulas/mathematics/middle-school/6k6epemxei0igaktrm2pw36fy23c4xckrh.png)

Subtract the resulting equations
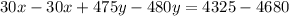
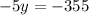
Divide both sides by -5
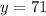
Substitute
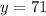 in (1)
in (1)

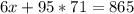
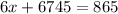
Collect like terms


Divide both sides by 6

The solution is correct but does not represent real life situation because x should be positive