Question

I really need help plsss!!!

Answer 1

(a)

`f(x) = \left \{ {{5x;\ 0<x\le 5} \atop {5x-5; x > 5}} \right.`

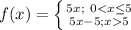
(b) Rate = 5

(c) Rate = 5

Explanation:

Given

The attached graph

Solving (a): The piece-wise function

For the first line

Calculate the slope of the first line

`m = (y_2 - y_1)/(x_2 - x_1)`

Where

`(x_1,y_1) = (0,0)`

`(x_2,y_2) = (5,25)`

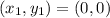
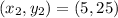
So, the slope is:

`m = (25 - 0)/(5 - 0)`

`m = (25)/(5)`

`m = 5`

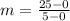
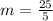
The equation is then calculated as:

`y = m(x - x_1) + y_1`

Where:
`m = 5` and
`(x_1,y_1) = (0,0)`

`y = 5(x - 0) + 0`

`y = 5(x) + 0`

`y = 5x`

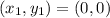
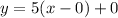
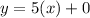
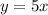
So, the function of the first line is:

`f(x) = 5x;\ 0 < x \le 5`

For the second line

Calculate the slope of the second line

`m = (y_2 - y_1)/(x_2 - x_1)`

Where

`(x_1,y_1) = (5,20)`

`(x_2,y_2) = (9,40)`

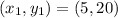
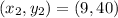
So, the slope is:

`m = (40 - 20)/(9 - 5)`

`m = (20)/(4)`

`m = 5`

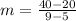
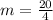
The equation is then calculated as:

`y = m(x - x_1) + y_1`

Where:
`m = 5` and
`(x_1,y_1) = (5,20)`

`y = 5(x - 5) + 20`

`y = 5x - 25 + 20`

`y = 5x -5`

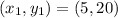
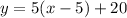
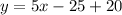
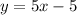
So, the function of the second line is:

`f(x) = 5x -5;\ x>5`

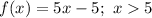
Hence, the piece-wise function is:

`f(x) = \left \{ {{5x;\ 0<x\le 5} \atop {5x-5; x > 5}} \right.`

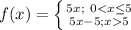
Solving (b): The rate at which customers that buy up to 5lb buy.

`x = 5`

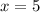
This question implies that, we determine the rate at which this customer buys.

For
`x = 5`

`f(x) = 5x;\ 0 < x \le 5`

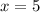
The slope of the above function is 5.

So, this customer buys at the rate of $5 per lb

Solving (b): The rate at which customers that buy more than 5lb buy the extra pounds above 5lb.

For
`x > 5`

`f(x) = 5x -5;\ x>5`

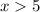
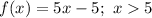
Assume x = 6

`f(6) = 5 * 6 - 5`

`f(6) = 30 - 5`

`f(6) = 25`

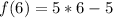
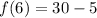
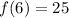
Express as 20 + 5

`f(6) = 20 + 5 * 1`

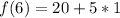
Assume x = 7

`f(7) = 5 * 7 - 5`

`f(7) = 35 - 5`

`f(7) = 30`

`f(7) = 20 + 10`

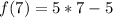
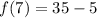
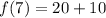
Express 10 as 5 * 2

`f(7) = 20 + 5 * 2`

So, we have:

`f(6) = 20 + 5 * 1`

`f(7) = 20 + 5 * 2`

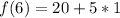
This can be generalized as:

`f(x) = 20 + 5(x - 5)`

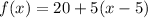
For the above functions;

20 represents the amount they buy the first 5

5 represents the rate at which they buy extra pounds above 5

Hence, they buy the extra pounds at the rate of $5 per pound