Question

Use the linear combination method to solve the system of equations. Please explain each step of your solution

2x - 3y = 13
x + 2y = -4

Answer 1

(2, -3)

Solve the equation

`\left \{ {{2x-3y=13} \atop {x+2y=-4}} \right.`

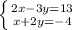
Rearrange like terms to the same side of the equation

`\left \{ {{2x-3y=13} \atop {x=-4-2y}} \right.`

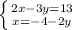
Substitute into one of the equations

`2(-4-2y)-3y=13`

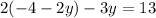
Apply the Distributive Property

`-8-4y-3y=13`

Combine like terms

`-8-7y=13`

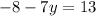
Rearrange variables to the left side of the equation

`-7y=13+8`

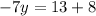
Calculate the sum or difference

`-7y=21`

Divide both sides of the equation by the coefficient of variable

`y=-(21)/(7)`

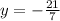
Cross out the common factor

`y=-3`

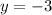
Substitute into one of the equations

`x=-4-2*(-3)`

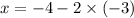
Calculate

`x=2`

The solution of the system is

`\left \{ {{x=2} \atop {y=-3}} \right.`

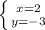
I hope this helps you

:)