Answer:
Train A is travelling at a speed of 12.857 miles per hour and train B at a speed of 9.643 miles per hour.
Explanation:
Let suppose that train A begins in position
 and the train B in position
and the train B in position
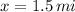 , if
, if
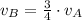 and both trains move at constant speed, then we have the following kinematic equations:
and both trains move at constant speed, then we have the following kinematic equations:
Train A
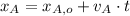 (1)
(1)
Train B
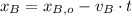 (2)
(2)
If both trains meet each other, then
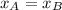 . If we know that
. If we know that
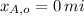 ,
,
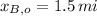 ,
,
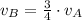 and
and
 , then we have the following expression:
, then we have the following expression:
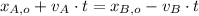
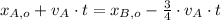
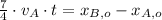
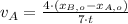

Then, the speed of the train B is:
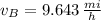
Train A is travelling at a speed of 12.857 miles per hour and train B at a speed of 9.643 miles per hour.