Question

Two long straight parallel lines, #1 and #2, carry uniform positive linear charge densities. The charge density on line #2 is twice as great as the charge density on line #1. The locus of points where the electric field due to these lines is zero is

along a line between the lines closer to line #2 than line #1.


at a point midway between the lines.


along a line between the lines closer to line #1 than line #2.


along a line perpendicular to lines #1 and #2.

Answer 1

The locus of points where the electric field due to these lines is zero is;

Along the line between the lines closer to line#1 than line #2

Step-by-step explanation:

The charges on the parallel lines #1 and #2 = Positive linear charge

The charge density on line #2, λ₂ = 2 × The charge density on line #1, λ₁

Therefore, we have;

λ₂ = 2 × λ₁

Electric field strength, E, is given as follows;

`E = (\lambda)/(2\cdot \pi\cdot \epsilon_0\cdot d)`

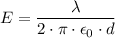
Therefore;

`E_1 = (\lambda_1)/(2\cdot \pi\cdot \epsilon_0\cdot d)`

`E_2 = (\lambda_2)/(2\cdot \pi\cdot \epsilon_0\cdot d) = 2 *(\lambda_1)/(2\cdot \pi\cdot \epsilon_0\cdot d)`

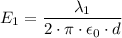
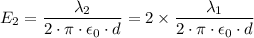
E₂ = 2·E₁

E₂/2 = E₁

E₂/(2·d) = E₁/d

The strength of the electric field at a given distance from line #2 is 2 times the strength of the electric field from line #1 at the same distance

Therefore the strength of the electric field will be the same at a point twice the distance from line #2 than from line #1 which is a point closer to line #1 than line #2.