Answer:
The coefficient of performance of the refrigerator is 2.251.
Step-by-step explanation:
In this case, the coefficient of performance of the refrigerator (
 ), no unit, is equal to the ratio of the heat rate received from the water to the power needed to work, that is:
), no unit, is equal to the ratio of the heat rate received from the water to the power needed to work, that is:
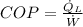 (1)
(1)
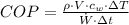 (2)
(2)
Where:
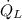 - Heat rate received from the water, in watts.
- Heat rate received from the water, in watts.
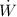 - Power, in watts.
- Power, in watts.
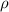 - Density of water, in kilograms per cubic meter.
- Density of water, in kilograms per cubic meter.
 - Volume of water, in cubic meters.
- Volume of water, in cubic meters.
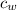 - Specific heat of water, in joules per kilogram-degree Celsius.
- Specific heat of water, in joules per kilogram-degree Celsius.
 - Temperature change, in degrees Celsius.
- Temperature change, in degrees Celsius.
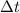 - Cooling time, in seconds.
- Cooling time, in seconds.
If we know that
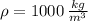 ,
,
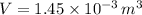 ,
,
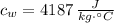 ,
,
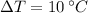 ,
,
 and
and
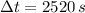 , then the coefficient of refrigeration of the refrigerator is:
, then the coefficient of refrigeration of the refrigerator is:
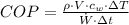

The coefficient of performance of the refrigerator is 2.251.