Answer:

Explanation:
We are given this proportion:
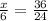
We want to solve for x, so we must isolate the variable using inverse operations.
It is being divided by 6. The inverse of division is multiplication, so we multiply both sides of the proportion by 6.
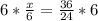
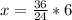
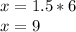
Another way to solve is with cross multiplication. Multiply the first numerator by the second denominator, then the first denominator by the second numerator.
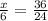
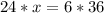
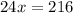
The variable is being multiplied by 24. The inverse of multiplication is division, so we divide both sides of the equation by 24.
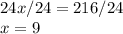
The value of x that makes this proportion true is 9.