Answer:
The required interest rate would be of 3.4% a year.
Explanation:
The amount of money earned in compound interest, after t years, is given by:
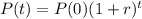
In which P(0) is the initial investment and r is the interest rate, as a decimal.
Peyton is going to invest $440 and leave it in an account for 5 years.
This means that
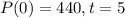
So
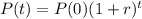
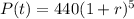
What interest rate, to the nearest tenth of a percent, would be required in order for Peyton to end up with $520?
This is r for which P(t) = 520. So
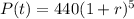
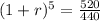
![\sqrt[5]{(1+r)^5} = \sqrt[5]{(52)/(44)}](https://img.qammunity.org/2022/formulas/mathematics/college/mhp88j49gupkt9sxftwoyfo0r6e1idbfay.png)
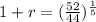
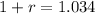
Then
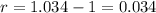
The required interest rate would be of 3.4% a year.