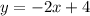Question

Write an equation in slope and intercept form of the line (0,4) and (2,0)

Answer 1

y = -2x + 4

Explanation:

1) First, find the slope of the line between the two points. Use the slope formula
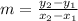`m = (y_2-y_1)/(x_2-x_1)` and substitute the x and y values of (0,4) and (2,0) into it. Then, solve:

`m = ((0)-(4))/((2)-(0)) \\m =(0-4)/(2-0) \\m = (-4)/(2) \\m = -2`

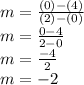
So, the slope is -2.

2) Now, identify the y-intercept of the line, or the point at which the line intersects the y-axis. All points on the y-axis have an x-value of 0. We're told that the line intersects (0,4), and that point has an x-value of 0. Thus, it must be the y-intercept.

3) With the found information, write the equation of the line in slope-intercept form, represented by the equation
`y = mx + b`. The
`m` , or the coefficient of the x-term, represents the slope. So, substitute -2 in its place. The
`b` represents the y-intercept of the line, so substitute 4 in its place. This gives the following answer:

`y = -2x + 4`