Answer:
108
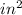
Explanation:
This polygon consists of two rectangles, one in the top-right hand corner and another in the bottom. To divide these two rectangles, imagine a line extended from the central horizontal line in the polygon. As I work the problem, remember that the formula for the area of a rectangle is
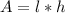 , where A is the area of the rectangle, l the length of the rectangle (one of the sides), and h is the height of the rectangle (the length of a side perpendicular to l).
, where A is the area of the rectangle, l the length of the rectangle (one of the sides), and h is the height of the rectangle (the length of a side perpendicular to l).
Starting with the top-right rectangle:
We already know one of the sides of the rectangle is 4 in, so let's imagine this to be h, because it is the height of the rectangle. Finding l is more complicated. We know the length of the larger rectangle is 12 in (more on that later) and the 6 in length is the part of the length that is not a part of the top-right rectangle. This, since the vertical line all the way to the right is straight, the length is the difference between these two lengths, or:
 in
in
Now that we know l and h, we can evaluate the area of the first rectangle, which is
 in^2
in^2
Now for the bottom rectangle:
We know both l and h for this rectangle, which are 12 in and 7 in respectively. Using the area formula for a rectangle above:
 in^2
in^2
Since the polygon contains both rectangles, its area is the sum of the areas of the two rectangles. Thus,
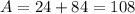 in^2
in^2
QED