Answer:
b, c and e
Explanation:
Given
See attachment for functions
Required
Select all statements that contain both functions
First, calculate the rate (slope) of both functions.
This is calculated as:

For Alec's
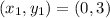
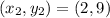
So, the slope is:
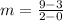
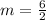
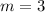
For Beth's
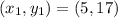
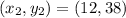
So, the slope is:
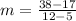
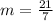
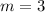
Beth and Rec have the same slope. Hence, they save at the same rate.
Next, determine the amount they started with.
This implies that we calculate y when x = 0
From Alec's graph.
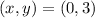
Alec's initial savings is 3
For Beth's savings, we make use of slope formula.

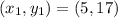
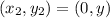
So, we have:
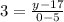
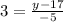

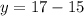
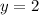
Beth's initial savings is 2
Hence, Alec started with more money
Next, is to determine who has more money after 10 days.
Since they save at the same rate, the difference in their savings after any number of days will be the difference in their initial savings.
The difference (d) is calculated as:

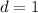
Hence, Alec will have $1 more than Beth after 10 days