Question

A field researcher is gathering data on the trunk diameters of mature pine and spruce trees in a certain area. The following are the results of his random sampling: Pine Trees Spruce Trees Sample Size 40 80 Mean trunk diameter (cm) 35 30 Population variance 160 160 Using a .10 significance level, can he conclude that the average trunk diameter of a pine tree is greater than the average diameter of a spruce tree

Answer 1

As the pvalue of the test is 0.02097 < 0.1, using a .10 significance level, he can conclude that the average trunk diameter of a pine tree is greater than the average diameter of a spruce tree.

Explanation:

Using a .10 significance level, can he conclude that the average trunk diameter of a pine tree is greater than the average diameter of a spruce tree

The null hypothesis is that they are equal(that is, subtraction between the means is 0), while the alternate hypothesis is that they are greater(that is, subtraction between the means is larger than 0). So

`H_0: \mu_1 - \mu_2 = 0`

`H_a: \mu_1 - \mu_2 > 0`

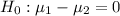
In which
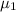`\mu_1` is the mean pine trees height while
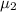`\mu_2` is the mean spruce trees height.

The test statistic is:

`z = (X - \mu)/(s)`

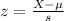
In which X is the sample mean,
`\mu` is the value tested at the null hypothesis and s is the standard error.

0 is tested at the null hypothesis:

This means that
`\mu = 0`

Mean trunk diameter (cm) 35 30:

Pine trees - 35

Spruce trees - 30

The sample mean is given by the subtraction of the means. So

`X = 35 - 30 = 5`

Sample Size 40 80

Population variance 160 160

This means that the standard error for each sample is given by:

`s_1 = (√(160))/(√(40)) = 2`

`s_1 = (√(160))/(√(80)) = √(2)`

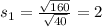
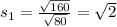
The standard error of the difference is the square root of the sum squared of the standard error of each sample.

`s = \sqrt{2^2 + (√(2))^2} = √(6) = 2.4495`

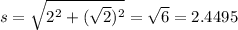
Test statistic:

`z = (X - \mu)/(s)`

`z = (5 - 0)/(2.4495)`

`z = 2.04`

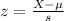
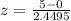
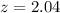
Pvalue of the test:

Probability of a sample mean larger than 5, which is 1 subtracted by the pvalue of Z when X = 5.

Looking at the z-table, z = 2.04 has a pvalue of 0.9793.

1 - 0.9793 = 0.0207

As the pvalue of the test is 0.02097 < 0.1, using a .10 significance level, he can conclude that the average trunk diameter of a pine tree is greater than the average diameter of a spruce tree.