Question

A wheel rotates about a fixed axis with an initial angular velocity of 24 rad/s. During a 4 s interval the angular velocity decreases to 14 rad/s. Assume that the angular acceleration is constant during the 4 s interval. How many radians does the wheel turn through during the 4 s interval

Answer 1

`\theta=76\ rad`

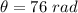
Step-by-step explanation:

Hoven that,

Initial angular velocity of the wheel = 24 rad/s

Final angular velocity = 14 m/s

Time, t = 4 s

We need to find how many radians does the wheel turn through during the 4 s interval. Let the displacement is
`\theta`. Using second equation of rotational kinematics to find it such that,

`\theta=\omega_i t+(1)/(2)\alpha t^2`

Where

`\alpha` is angular acceleration

`\alpha =(\omega_f-\omega_i)/(t)\\\\\alpha =(14-24)/(4)\\\\\alpha =-2.5\ rad/s^2`

So,

`\theta=24* 4+(1)/(2)* (-2.5)* 4^2\\\\\theta=76\ rad`

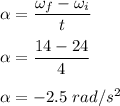
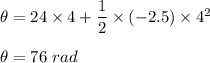
So, it will turn 76 radian during the 4 s interval.