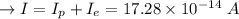Question

Suppose that a particle accelerator is used to move two beams of particles in opposite directions. In a particular region, electrons move to the right at 4910 m/s and protons move to the left at 2583 m/s. The particles are evenly spaced with 0.0758 m between electrons and 0.0577 m between protons. Assuming that there are no collisions and that the interactions between the particles are negligible, what is the magnitude of the average current in this region

Answer 1

The answer is "
`17.28 * 10^(-14)\ A`"

Step-by-step explanation:

Calculating the number of electrons passing in per second:

`\to n_e = (4910)/(0.0758) = 6.4 * 10^5`

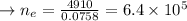
Calculating the current in electron:

`\to I_e = 6.4 * 10^5 * 1.6 * 10^(-19) = 10.24 * 10^(-14)\ A`

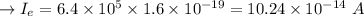
Calculating the number of protons passing in per second:

`\to n_p = (2583)/(0.0577) = 4.4 * 10^(4)`

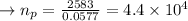
Calculating the current in proton:

`\to I_p = 4.4 * 10^(4) * 1.6 x 10^(-19) = 7.04 * 10^(-14) \ A`

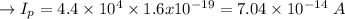
Calculating the total current:

`\to I = I_p + I_e = 17.28 * 10^(-14)\ A`