Question

If you buy a motorcycle for $12,500 in 2015 and it depreciates (shrinks) in value by 15.5% every year, how much will it be worth in 2020?

A) $10,562.50
B) $1718.33
C) $6732.90
D) $5385.10

Answer 1

D) $5385.10

Explanation:

Exponential equation of decay:

The exponential equation for the decay of an amount after t years is given by:

`A(t) = A(0)(1-r)^t`

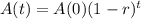
In which A(0) is the initial amount and r is the decay rate, as a decimal.

If you buy a motorcycle for $12,500 in 2015 and it depreciates (shrinks) in value by 15.5% every year

This means that
`A(0) = 12500, r = 0.155`

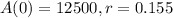
So

`A(t) = A(0)(1-r)^t`

`A(t) = 12500(1-0.155)^t`

`A(t) = 12500(0.845)^t`

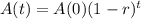
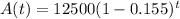
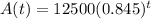
How much will it be worth in 2020?

2020 is 2020 - 2015 = 5 years after 2015, so this is A(5). So

`A(5) = 12500(0.845)^5 = 5385.10`

The correct answer is given by option D.