Water is the Adjacent of this right triangle. 350 yards is the length of the Hypotenuse. We have given angle B, 40 degrees.
_________________________________________________________
According to SOH CAH TOA, we use CAH (cosineΘ = adjacent/hypotenuse).
- Θ = 40
- hyoptenuse = 350
- adjacent = x
cosine40 = x/350
[multiply both sides by 350]
350 · cosine40 = x
[put calculator in degree mode]
x ≈ 268 yards
So, the distance across the water from sailboat to the island is 268 yards.
_________________________________________________________
Let's use pythagorean theorem since we already know the adjacent and hypotenuse to find the opposite (opposite is also known as height).
a^2 + b^2 = c^2
- a = ? (opposite or height)
- b = 268 (adjacent)
- c = 350 (hypotenuse)
a^2 + (268)^2 = (350)^2
a^2 + 71824 = 122500
a^2 = 122500 - 71824
a^2 = 50676
[square both sides]
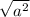 =
=
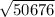
a =
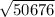
a ≈ 225
So, the height, opposite, of the lighthouse is 225 yards.
_________________________________________________________
ANSWER:
a) 268 yards
b) 225 yards