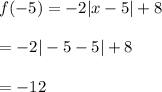Answer:
Domain: set of possible input values (x-values)
Range: set of output values (y-values)
The range for the function
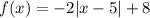 is:
is:
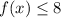
(5, 8) is the vertex of the line
Because |x - 5| is absolute (so always positive) and it is multiplied by -2,
-2|x - 5| will always be negative unless x = 5 (when it will be zero). Therefore, the max y-value is 8.
However, your question actually asked what the function is when x = -5: