Question

Prove that underroot 3-underroot 5 is irrational.​
Please quickly I need this answer

Answer 1

See Explanation

Explanation:

Let us assume that
`\sqrt 3 - \sqrt 5` is a rational number.

So, it can be expressed in the form of
`(p)/(q)` where p and q are integers.

`\therefore \sqrt 3 - \sqrt 5=(p)/(q)`

`\therefore \sqrt 3 =(p)/(q)+ \sqrt 5`

`\therefore \sqrt 3 =(p+q\sqrt 5)/(q)`

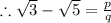
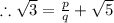
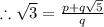
Squaring both sides:

`(\sqrt 3) ^2 =\bigg((p+q\sqrt 5)/(q)\bigg ) ^2`

`\therefore 3 =(p^2 +5q^2 +2\sqrt 5 pq)/(q^2 )`

`\therefore 3q^2 ={p^2 +5q^2 +2\sqrt 5 pq}`

`\therefore 0 = p^2 +5q^2 -3q^2 +2\sqrt 5 pq`

`\therefore - 2\sqrt 5 pq=p^2 +2q^2`

`\therefore \sqrt 5 = \bigg ((p^2 +2q^2)/(-2pq) \bigg)`

`\therefore \sqrt 5 = - \bigg((p^2 +2q^2)/(2pq) \bigg)`

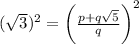
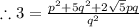
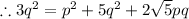
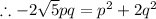
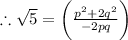
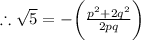
Since, p and q are integers so
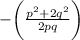`- \bigg((p^2 +2q^2)/(2pq) \bigg)` is rational.

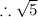`\therefore \sqrt 5` is also rational.

But it contradicts the fact that
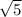`\sqrt 5` is irrational.

Hence, our assumption that
`\sqrt 3 - \sqrt 5` is rational is wrong.

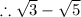`\therefore \sqrt 3 - \sqrt 5` is irrational.