Using the shell method, the volume of the solid generated by revolving the shaded region about the y-axis, given by
 . Thus, the correct answer is A.
. Thus, the correct answer is A.
To find the volume of the solid generated by revolving the shaded region about the y-axis using the shell method, we'll integrate along the y-axis.
The equation
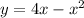 can be rewritten as
can be rewritten as
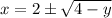 . To find the limits of integration, we need to determine the points where the curves intersect:
. To find the limits of integration, we need to determine the points where the curves intersect:
![\[4x - x^2 = 0\]](https://img.qammunity.org/2022/formulas/mathematics/college/wdrpg2t4oxjqjsooz77s9lswl53z0arbnu.png)
Factoring out x, we get
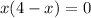 . So,
. So,
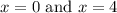 are the points of intersection.
are the points of intersection.
Now, the radius of the shell is the distance from the y-axis to the curve, which is x. The height of the shell is the differential change in y, denoted as dy.
The volume element of a shell is
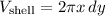 .
.
Now, integrate
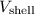 from
from
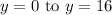 (the square of
(the square of
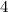 ):
):
![\[V = \int_(0)^(16) 2\pi x \, dy\]\[V = \int_(0)^(16) 2\pi(2 + √(4 - y)) \, dy\]](https://img.qammunity.org/2022/formulas/mathematics/college/vcof3lg8cj96k3il6nk5enavtmu9vt9dni.png)
Evaluate this integral to find the volume.
![\[V = \int_(0)^(16) 2\pi(2 + √(4 - y)) \, dy = (128)/(3)\pi\]](https://img.qammunity.org/2022/formulas/mathematics/college/hpsx8ege30z64vwhttjgywgf1dftl5y6ee.png)
Therefore, the correct answer is A.
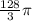 .
.