Question

Find the lengths of the legs.
(NO LINKS)

Answer 1

Given:

The figure of a right angle triangle with hypotenuse 10 and two angles
`45^\circ, 90^\circ`

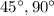
To find:

The lengths of the legs.

Solution:

Two angles are given. By using the angle sum property, the third angle is:

Third angle =
`180^\circ-45^\circ- 90^\circ`

=
`45^\circ`

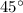
Base angles are equal it means the given triangle is an isosceles right triangle. So, the lengths of both legs are equal.

Let x be the lengths of both legs. Then by using Pythagoras theorem, we get

`Hypotenuse^2=leg_1^2+leg_2^2`

`(10)^2=x^2+x^2`

`100=2x^2`

`(100)/(2)=x^2`

`50=x^2`

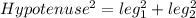
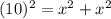
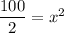
Taking square root on both sides, we get

`\pm √(50)=x`

`\pm 5√(2)=x`

`\pm 5√(2)=x`

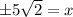
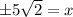
The side length cannot be negative. So, the only value of x is
`5√(2)`.

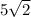Therefore, the length of the both legs is
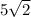`5√(2)` units.