Question

A vertical piston-cylinder device initially contains 0.1 m^3 of air at 400 K and 100 kPa. At this initial condition, the piston is resting on a stop. The piston-cylinder device is connected to a supply line with air at 400 K and a pressure of 500 kPa. The valve between the supply line and the piston-cylinder device is opened and is left open until the pressure in the piston-cylinder device reaches 500 kPa. The piston is observed to start moving when the pressure in the cylinder is 200 kPa and the piston continues to rise until it reaches a second stop. At the second stop, the piston-cylinder volume is 0.2 m^3 . The final temperature and pressure in the piston-cylinder device are 440 K and 500 kPa, respectively. Determine the heat transfer to or from the piston-cylinder device for the filling process Determine heat transfer (kJ) during the entire process.

Answer 1

The calculation of heat transfer in a piston-cylinder device requires applying thermodynamic principles and knowledge of specific heat capacities, considering the work done by the gas under changes in pressure, volume, and temperature.

Step-by-step explanation:

The student's question involves the calculation of heat transfer to or from a piston-cylinder device that experiences a change in pressure, volume, and temperature of a contained gas—specifically air. This process is done by opening a valve to a supply line with higher pressure air. To determine the heat transfer for the filling process and the entire process, principles from thermodynamics, such as the first law of thermodynamics, and equations of state for an ideal gas, need to be applied. The heat transfer can be found by using the specific heat capacities of air at constant volume (if the process is isochoric) or at constant pressure (if the process is isobaric) and considering the work done by the system. Without detailed information about the specific heat capacities and whether heat is added or removed, a precise numerical answer cannot be provided.

Answer 2

`Q=-38.15kJ`

Step-by-step explanation:

From the question we are told that

Piston-cylinder initial Volume of air
`v_1=0.1 m^3`

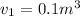
Piston-cylinder initial temperature
`T_1=400k`

Piston-cylinder initial pressure
`P_1= 100kpa`

Supply line temperature
`T_s=400k`

Supply line pressure
`P_s= 500kpa`

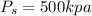
Valve final pressure
`P_v=500kpa`

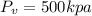
Piston movement pressure
`P_m=200kpa`

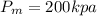
Piston-cylinder final Volume of air
`v_2=0.2 m^3`

Piston-cylinder final temperature
`T_2=440k`

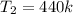
Piston-cylinder final pressure
`P_2= 500kpa`

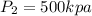
Generally the equation for conservation of mass is mathematically given by

`Q=m_2 \mu_2-m_1 \mu_1 +W-(m_2-m_1)h`

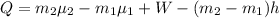
where

Initial moment

`m_1=(p_1 V_1)/(RT_1)`

`m_1=(100*0.1)/(0.287*400)`

`m_1=8.7*10^-^2kg`

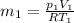
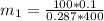
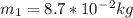
Final moment

`m_2=(p_2 V_2)/(RT_2)`

`m_1=(500*0.3)/(0.287*440)`

`m_1=79*10^(-2)kg`

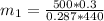
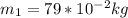
Work done by Piston movement pressure

`W=Pd`

`W=P(v_2-v_1)`

`W=200(0.2-0.1))`

`W=20000J`

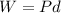
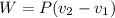
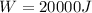
Heat function

`h=cT_1`

`h=1.005(400)`

`h=402`

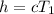
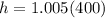
Therefore

`Q=(0.792*0.718(440)-0.0871*0.718(400)+20-(0.792-0.087)*402))`

`Q=-38.15kJ`

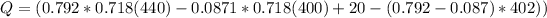
It is given mathematically that the system lost or dissipated Heat of

`Q=-38.15kJ`