Answer:
C. (p - 6q)(p² + 6pq + 36q²)
Explanation:
Equation at the end of step 1
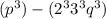
Trying to factor as a Difference of Cubes:
Factoring: p^3 - 216q^3
Theory: A difference of two perfect cubes, a^3 - b^3 can be factored into
(a-b) • (a^2 +ab +b^2)
Proof:
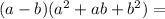
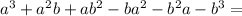
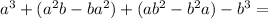

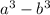
Check: 216 is the cube of 6
Check: p^3 is the cube of p^1
Check: q^3 is the cube of q^1
Factorization is:
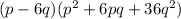
Trying to factor a multi variable polynomial :
Factoring: p^2 + 6pq + 36q^2
Try to factor this multi-variable trinomial using trial and error
Factorization fails
Final Result:
(p - 6q)(p² + 6pq + 36q²)