Answer:
The statement about the p-value obtained from the data and the conclusion of the significant test that is true is option D
D. The p-value is greater than 0.10, therefore, there is no significant difference in mean search times on the two Web sites
Explanation:
From the question, the statistics student wants to compare the mean times needed to access flight information for two airlines
Let 'A' represent one of the airlines and let 'B' represent the other airline
In the statistics hypothesis test we have;
The mean time to access airline A's website,
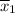 = 2.5 minutes
= 2.5 minutes
The standard deviation to access airline A's website, s₁ = 0.8 minutes
The mean time to access airline B's website,
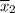 = 2.1 minutes
= 2.1 minutes
The standard deviation to access airline B's website, s₂ = 1.1 minutes
The t-test is given as follows;
The null hypothesis is H₀; μ₂ - μ₁ = 0
The alternative hypothesis is H₀; μ₂ - μ₁ ≠ 0
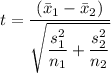
The critical-t at n₁ + n₂ - 2 = 20 + 20 - 2 = 38 degrees of freedom is therefore, from a graphing calculator, the critical-t = ±2.734 and the probability = 0.197;
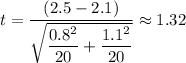
Therefore, given that the test statistic is smaller than the critical-t, and the p-value is greater than the significance level of 0.01 and is also larger than 0.10, therefore, there is no significant difference in mean search times on the two Web sites.