Answer:
The best point estimate of the population proportion p is 0.0251.
The 99% confidence interval for the proportion of adverse reactions is (0.0191, 0.0311).
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.

In which
z is the zscore that has a pvalue of
 .
.
In clinical trials, among 4547 patients treated with the drug, 114 developed the adverse reaction of nausea.
This means that
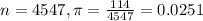
The best point estimate of the population proportion p is 0.0251.
99% confidence level
So
 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
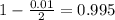 , so
, so
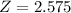 .
.
The lower limit of this interval is:
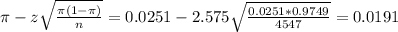
The upper limit of this interval is:
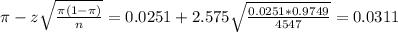
The 99% confidence interval for the proportion of adverse reactions is (0.0191, 0.0311).