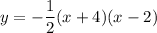Question

Write a quadratic function in the given form whose graph satisfies the given condition.

passes through (-4, 0), (2, 0), & (6, -20) in intercept form.
pls dont just steal points away...i rly need help with this...can you provide work to if not thats ok

Answer 1

`\displaystyle y=-(1)/(2)(x+4)(x-2)`

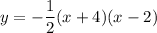
Explanation:

We want to find a quadratic that passes through the points:

`(-4, 0), \, (2, 0), \text{ and } (6, -20)`

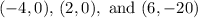
In intercept form.

First, note that the first two given points are the x-intercepts of our quadratic. Intercept or factored form is given by:

`y=a(x-p)(x-q)`

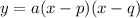
Where p and q are the x-intercepts, and a is the leading coefficient.

So, we will substitute -4 and 2 for p and q:

`y=a(x-(-4))(x-(2))`

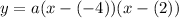
Simplify:

`y=a(x+4)(x-2)`

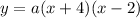
Next, the third point (6, -20) tells us that y = -20 when x = 6. So:

`(-20)=a(6+4)(6-2)`

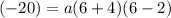
Solve for a:

`-20=10(4)a\Rightarrow 40a=-20`

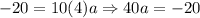
Thus:

`\displaystyle a=(-20)/(40)=-(1)/(2)`

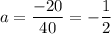
Hence, our equation in intercept from is:

`\displaystyle y=-(1)/(2)(x+4)(x-2)`