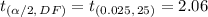Answer:
The t-score to be used to find the 95% confidence interval for the population mean is;
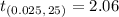
Explanation:
The t-score which is representative of a point with n - k (n, and k represents the sample size and the number of groups in the test respectively) degrees of freedom from the Student's T-distribution is a standard test statistic obtained by applying a T-test
Therefore, the t-score used for a confidence interval is obtained by by making use of the the following information;
The number of degrees of freedom = n - k
The confidence level in the confidence interval
The parameters given in the question are;
The number of pizza orders in the sample, n = The sample size = 26
The number of samples used in the estimate, k = 1 group of 26 randomly sampled pizza
The level of confidence (the probability) that the interval contains the population mean = 95%
Therefore, the significance level, α = 1 - 95% = 1 - 95/100 = 0.05
∴ The α-level for the upper and lower limit of the interval are α/2 = 0.05/2 = 0.025 long
The degrees of freedom, df = n - k
∴ For the estimation, DF = 26 - 1 = 25
The t-statistic is therefore the t-value at the intersection of the row for DF = 25 and the 0.025 (one-tail) column (0.025 two-tail column) which gives;